ETC Main Formula

Very useful informations given by an ETC are the expected number of counts for an observed object and contribute of the sky, and the signal to noise ratio. The ETC gives also other informations which are usually instrument specific and so we prefer to describe them in other documents.

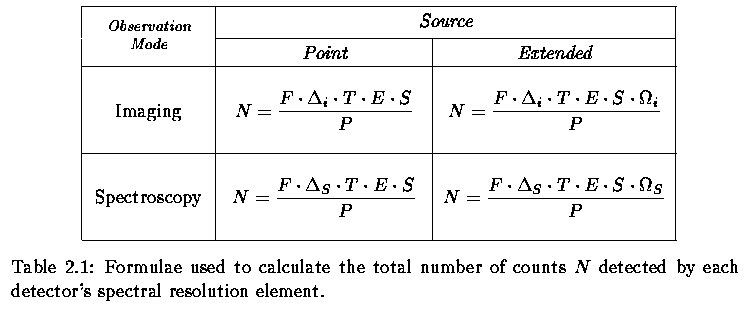
The main formulae used to evaluate the expected number of counts and the signal to noise ratio are given in the following table:

where N is the number of electrons per bin, F is the incident flux in $W\slash m^{2}\slash \mu m$, $\Delta _{S}$, in spectroscopy, is the spectral bin in $\mu m\slash bin$, $\Delta _{i}$, in imaging, is the filter band width $\mu m$, T the total exposure time in seconds, E the efficiency, S the telescope surface in m², $\Omega_{S}$ is the solid angle subtended by the integration element (in Spectroscopy), $\Omega_{i}$ is the solid angle subtended by the integration element (in imaging), P the energy of one photon. The resulting dimension of N is $[N]=e^{-}\slash bin$.

 

To calculate the signal to noise ratio the ETC uses the formulae:

$$\frac{S}{N}=\frac{N_{Obj}}{\sqrt{N_{Obj}+n_{bin}\cdot N_{Sky}+ n_{bin}\cdot RON^{2}+n_{pix}\cdot DARK\cdot T}}$$ (2.1)

or in the infrared:

$$\frac{S}{N}=\frac{N_{Obj}\cdot \sqrt{N\_DIT}} {\sqrt{N_{Obj}+... ..._{Sky}+ n_{bin}\cdot RON^{2}+2\cdot n_{bin}\cdot DARK\cdot T}}$$ (2.2)

where

$$n_{pix}=2\times\frac{seeing}{pix\_scale}$$ (2.3)

is the number of spatial pixels over which it is integrated the signal ^2.1, where seeing is the seeing expressed in arcseconds; ${\mbox pix\_scale}$ is the dimension of one pixel in arcseconds; N_Obj is the total number of counts per spectral bin coming from the observed object (point source or extended one); N_Sky is the similar term due to the sky; RON is the contribute to the detected counts due to the Read Out Noise; DARK is the similar term due to the dark counts.

 

Table 2.2: Table with summary definitions of the terms present in the formula used to calculate the number of expected counts and the signal to noise ratio..

simbol	units	meaning
NObj	$e^{-}\slash DIT$	object signal
NSky	$e^{-}\slash s\slash bin$	sky signal
DARK	$e^{-}\slash s\slash bin$	dark current
RON	$e^{-}\slash bin$	read out noise
npix		number of integration pixels to evaluate the RON contribute to S/N
nbin		number of integration bins to evaluate the DARK contribute to S/N
T	s	detector integration time
$N\_DIT$		number of detector integrations
$\Omega_{S}$	arcsec2	solid angle subtended by the integration element (in Spectroscopy)
$\Omega_{i}$	arcsec2	solid angle subtended by the integration element (in imaging)
$\Delta _{S}$	$\mu m\slash bin$	spectral bin (in Spectroscopy)
$\Delta _{i}$	$\mu m\slash bin$	filter band width (in imaging)

$$N_{0}=N\cdot DIT\Longrightarrow \left[N_{0}\right]=s$$

The parameters present in Table 2.1 are defined or from user specifications, the ETC front page input parameters, or by other sources of information (instrument known characteristics, physical relations, the database).

How we obtain each result is described in its own following section.